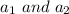Complete Question
The complete question is shown on the first uploaded image
Answer:
a
b can be written as a linear combination of
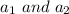
b
The values of
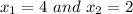
Explanation:
From the question we are told that
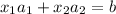
Where
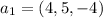 ,
,
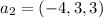 and
and
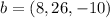
So
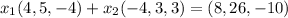

=>
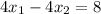
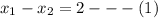
=>
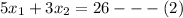
=>

Now multiplying equation 1 by 3 and adding the product to equation 2
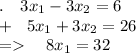
=>
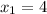
substituting
 into equation 1
into equation 1
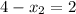
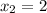
Now to test substitute
 into equation 3
into equation 3

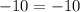
Since LHS = RHS then there exist values
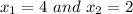 such that
such that
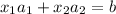
Hence b can be written as a linear combination of