Answer:
1) The null and alternative hypothesis are:
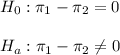
Subindex 1: 2012 population proportion and Subindex 2: 2010 population proportion.
2) The sample proportion for 2012 is p1=0.41.
The sample proportion for 2010 is p2=0.35.
3) There is enough evidence to support the claim that there is significant difference between the population proportions of the two years.
4) The 95% confidence interval for the difference between proportions is (0.016, 0.104).
Explanation:
This is a hypothesis test for the difference between proportions.
The claim is that there is significant difference between the population proportions of the two years.
Then, the null and alternative hypothesis are:
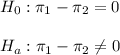
The significance level is 0.05.
The sample 1, of size n1=1000 has a proportion of p1=0.41.

The sample 2, of size n2=900 has a proportion of p2=0.35.
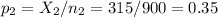
The difference between proportions is (p1-p2)=0.06.
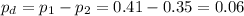
The pooled proportion, needed to calculate the standard error, is:

The estimated standard error of the difference between means is computed using the formula:
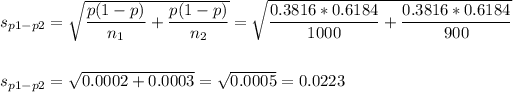
Then, we can calculate the z-statistic as:
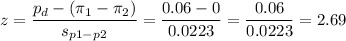
This test is a two-tailed test, so the P-value for this test is calculated as (using a z-table):
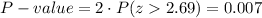
As the P-value (0.007) is smaller than the significance level (0.05), the effect is significant.
The null hypothesis is rejected.
There is enough evidence to support the claim that there is significant difference between the population proportions of the two years.
We want to calculate the bounds of a 95% confidence interval.
For a 95% CI, the critical value for z is z=1.96.
The margin of error, using the results previously calculated, is:
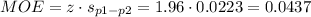
Then, the lower and upper bounds of the confidence interval are:

The 95% confidence interval for the difference between proportions is (0.016, 0.104).