Question

Suppose that it is known that the heights of male Shar Pei dogs are normally distributed with a mean of 19.5 inches and a standard deviation of 0.5 inches. In a random sample of 10 male Shar Pei dogs what is the probability that at least one is taller than 21 inches? Give your answer to 3 decimal places.

Answer 1

0.013 = 1.3% probability that at least one is taller than 21 inches

Explanation:

To solve this question, we need to understand the binomial distribution and the normal distribution.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

Normal probability distribution

When the distribution is normal, we use the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Proportion of male dogs taller than 21 inches:

A mean of 19.5 inches and a standard deviation of 0.5 inches. This means that
`\mu = 19.5, \sigma = 0.5`

This proportion is 1 subtracted by the pvalue of Z when X = 21.

`Z = (X - \mu)/(\sigma)`

`Z = (21 - 19.5)/(0.5)`

`Z = 3`

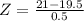
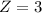
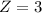`Z = 3` has a pvalue of 0.9987

1 - 0.9987 = 0.0013.

In a random sample of 10 male Shar Pei dogs what is the probability that at least one is taller than 21 inches?

0.0013 of the dogs are taller than 21 inches, so
`p = 0.0013`

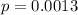
10 dogs, so
`n = 10`

Either no dog is taller than 21 inches, or at least one is. The sum of the probabilities of these events is 1. So

`P(X = 0) + P(X \geq 1) = 1`

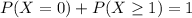
We want
`P(X \geq 1)`

`P(X \geq 1) = 1 - P(X = 0)`

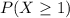
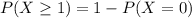
In which

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 0) = C_(10,0).(0.0013)^(0).(0.9987)^(10) = 0.987`

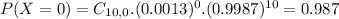
Then

`P(X \geq 1) = 1 - P(X = 0) = 1 - 0.987 = 0.013`

0.013 = 1.3% probability that at least one is taller than 21 inches