Answer:
the g's contributing term for the overall uncertainty of P is
![dP_g = [(dg)/(g)]](https://img.qammunity.org/2021/formulas/mathematics/college/248d3uutlijri52k1nrhqg1hlc3c21q8b4.png)
Explanation:
From the question we are told that
The pressure is
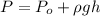
The first step in determining the uncertainty of P in by obtaining the terms in the equation contributing to it uncertainty and to do that we take the Ln of both sides of the equation
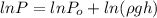
=>
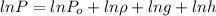
Then the next step is to differentiate both sides of the equation
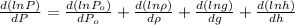
=>
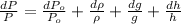
We asked to obtain the contribution of the term g to the uncertainty of P
This can deduced from the above equation as
![dP_g = [(dg)/(g)] P](https://img.qammunity.org/2021/formulas/mathematics/college/rkw6y25q1s9e908u19nsygv01j6ugsua0x.png)