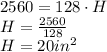Question

If the volume of the planter is 2,560 cubic inches,how tall is the planter

Answer 1

Height of Planter = H = 20 in²

Explanation:

As the planter is not given in the question, the question is incomplete.

First, lets complete the question by attaching the diagram related to this question.

(SEE THE ATTACHMENT)

Lets consider the right angled triangle that is formed at the top of the planter, as shown in the diagram attached.

The right angled triangle has a width of 16 inches and a heights of 16 inches as well.

We know that the Area of a triangle can be calculated by following formula:

`A=(1)/(2)\cdot(width)\cdot(height)`

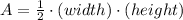
Substitute the values to find Area:

`Area = (1)/(2)\cdot16\cdot16\\Area = 128 in^2`

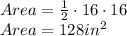
We also know that the Volume of a triangular prism is given by:

`Volume=(Area)\cdot(Height)`

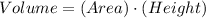
Where

Area = Area of the face = 128 in²

Height = Height of the planter = H

Volume = 2560 in³

Substitute the values and solve for H:

`2560=128\cdot{H}\\H=(2560)/(128)\\H=20in^2`