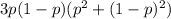Answer:
The probability that the series lasts exactly four games is
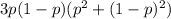
Explanation:
For each game, there are only two possible outcomes. Either team A wins, or team A loses. Games are played independently. This means that we use the binomial probability distribution to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.
We also need to know a small concept of independent events.
Independent events:
If two events, A and B, are independent, we have that:
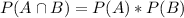
What is the probability that the series lasts exactly four games?
This happens if A wins in 4 games of B wins in 4 games.
Probability of A winning in exactly four games:
In the first two games, A must win 2 of them. Also, A must win the fourth game. So, two independent events:
Event A: A wins two of the first three games.
Event B: A wins the fourth game.
P(A):
A wins any game with probability p. 3 games, so n = 3. We have to find P(A) = P(X = 2).


P(B):
The probability that A wins any game is p, so P(B) = p.
Probability that A wins in 4:
A and B are independent, so:
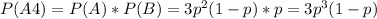
Probability of B winning in exactly four games:
In the first three games, A must win one and B must win 2. The fourth game must be won by 2. So
Event A: A wins one of the first three.
Event B: B wins the fourth game.
P(A)
P(X = 1).

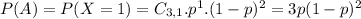
P(B)
B wins each game with probability 1 - p, do P(B) = 1 - p.
Probability that B wins in 4:
A and B are independent, so:
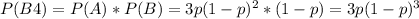
Probability that the series lasts exactly four games:
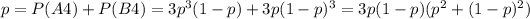
The probability that the series lasts exactly four games is