Question

Money Spent on Road Repair A politician wishes to compare the variances of the amount of money spent for road repair in two different counties. The data are given here. At α = 0.05, is there a significant difference in the variances of the amounts spent in the two counties? Use the P-value method.

County A County B
s1 = $11,596 s2 = $14,837
n1 = 15 n2 = 18
A. State the hypotheses and identify the claim
B. Find the critical value(s).
C. Compute the test value.
D. Make the decision.

Answer 1

(A) Null Hypothesis,
`H_0` :
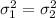`\sigma_1^(2) =\sigma_2^(2)` {means that there is no significant difference in the variances of the amounts spent in the two counties}

Alternate Hypothesis,
`H_A` :
`\sigma_1^(2) \\eq \sigma_2^(2)` {means that there is a significant difference in the variances of the amounts spent in the two counties}

(B) The F-table gives critical values of 0.359 and 2.781 for (14,17) degrees of freedom for the two-tailed test.

(C) The value of the F-test statistic is 0.611.

(D) We conclude that there is no significant difference in the variances of the amounts spent in the two counties.

Explanation:

We are given that a politician wishes to compare the variances of the amount of money spent on road repair in two different counties.

The data are given here below;

County A County B

s1 = $11,596 s2 = $14,837

n1 = 15 n2 = 18

Let
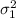`\sigma_1^(2)` = variances of the amounts spent in County A.

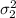`\sigma_2^(2)` = variances of the amounts spent in County B.

(A) So, Null Hypothesis,
`H_0` :
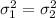`\sigma_1^(2) =\sigma_2^(2)` {means that there is no significant difference in the variances of the amounts spent in the two counties}

Alternate Hypothesis,
`H_A` :
`\sigma_1^(2) \\eq \sigma_2^(2)` {means that there is a significant difference in the variances of the amounts spent in the two counties}

The test statistics that would be used here Two-sample F-test statistics distribution;

T.S. =
`(s_1^(2) )/(s_2^(2) ) * (\sigma_2^(2) )/(\sigma_1^(2) )` ~
`F__n_1_-_1,_ n_2_-_1`

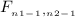
where,
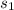`s_1` = sample standard deviation for County A = $11,596

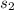`s_2` = sample standard deviation for County B = $14,837

`n_1` = sample size for County A = 15

`n_2` = sample size for County B = 18

(B) Now at 0.05 level of significance, the F-table gives critical values of 0.359 and 2.781 for (14,17) degrees of freedom for the two-tailed test.

(C) So, the test statistics =
`(11,596^(2) )/(14,837^(2) ) * 1` ~
`F__1_4,_ 1_7`

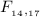
= 0.611

The value of the F-test statistic is 0.611.

Now, as we can see that our test statistics lie within the range of critical values of F, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region.

(D) Therefore, we conclude that there is no significant difference in the variances of the amounts spent in the two counties.