Answer:
a). ΔABC ~ ΔBDC
b). m(BD) = 6 units
Explanation:
If ΔABC ~ ΔBDC,
a). Ratio of the corresponding sides of both the triangles will be equal.
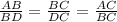

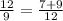

Since Ratio of the corresponding sides are same, therefore, both the triangles are similar.
b).
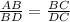
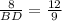
BD =
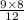
BD = 6