Question

A lake has a surface area of 800 square kilometers. What is the minimum length the shoreline could be

Answer 1

The minimum length of the shoreline is 100.26 km

Explanation:

We can start by assuming that the shape of the lake can be approximated to be a circle.

We need to understand that the length of the shoreline that we are looking for is the circumference of the circle.

From the area of the circle, we can get the radius as follows:

`Area = \pi r^(2)\\r = \sqrt{(Area)/(\pi)} \\r = \sqrt{(800)/(\pi)}= 15.96 km`

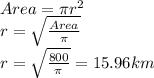
The circumference of the circle can be got using the formula

`circumference = 2\pi r`

`circumference = 2 * \pi * 15.96=100.26 km`

Therefore, the minimum length of the shoreline is 100.26 km