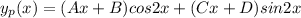Question

Match the following guess solutions ypyp for the method of undetermined coefficients with the second-order nonhomogeneous linear equations below.

A. yp(x)=Ax2+Bx+Cyp(x)=Ax2+Bx+C,
B. yp(x)=Ae2xyp(x)=Ae2x,
C. yp(x)=Acos2x+Bsin2xyp(x)=Acos⁡2x+Bsin⁡2x,
D. yp(x)=(Ax+B)cos2x+(Cx+D)sin2xyp(x)=(Ax+B)cos⁡2x+(Cx+D)sin⁡2x
E. yp(x)=Axe2x,yp(x)=Axe2x, and
F. yp(x)=e3x(Acos2x+Bsin2x)yp(x)=e3x(Acos⁡2x+Bsin⁡2x)
1. d2ydx2−5dydx+6y=e2xd2ydx2−5dydx+6y=e2x
2. d2ydx2+4y=−3x2+2x+3d2ydx2+4y=−3x2+2x+3
3. y′′+4y′+20y=−3sin2xy″+4y′+20y=−3sin⁡2x
4. y′′−2y′−15y=e3xcos2x

Answer 1

Explanation:

1 ) Given that

`(d^2y/dx^2) + 4y = x - x^2 + 20\\\\ (d^2y/dx^2) + 4y = - x^2 + x + 20`

For a non homogeneous part
`- x^2 + x + 20` , we assume the particular solution is

`y_p(x) = Ax^2 + Bx + C`

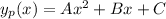
2 ) Given that

`d^2y/dx^2 + 6dy/dx + 8y = e^(2x)`

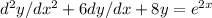
For a non homogeneous part
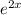`e^(2x)` , we assume the particular solution is

`y_p(x) = Ae^(2x)`

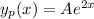
3 ) Given that

y′′ + 4y′ + 20y = −3sin(2x)

For a non homogeneous part −3sin(2x) , we assume the particular solution is

`y_p(x) = Acos(2x)+Bsin(2x)`

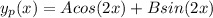
4 ) Given that

y′′ − 2y′ − 15y = 3xcos(2x)

For a non homogeneous part 3xcos(2x) , we assume the particular solution is

`y_p(x) = (Ax+B)cos2x+(Cx+D)sin2x`