Question

The American College Health Association produces the National College Health Assessment. The assessment indicates that the percentage of students who report having been diagnosed with depression has increased. Let’s assume that in 2010, 10% of students in one of the college campuses indicated that they had been diagnosed with depression, while in 2011, 15% of students in the same college campus indicated that they had been diagnosed with depression. The sample sizes were respectively 200 and 100.

Required:
At 0.01 level of significance, can we conclude that the proportion of college students who report having been diagnosed with depression has increased from 2010 to 2011? Make sure to state the null and the alternative hypotheses.

Answer 1

No. There is not enough evidence to support the claim that the proportion of college students who report having been diagnosed with depression has increased from 2010 to 2011.

The null and alternative hypothesis are:

`H_0: \pi_1-\pi_2=0\\\\H_a:\pi_1-\pi_2< 0`

(Subindex 1 for 2010 and subindex 2 for 2011)

Explanation:

This is a hypothesis test for the difference between proportions.

The claim is that the proportion of college students who report having been diagnosed with depression has increased from 2010 to 2011.

Then, the null and alternative hypothesis are:

`H_0: \pi_1-\pi_2=0\\\\H_a:\pi_1-\pi_2< 0`

The significance level is 0.01.

The sample 1 (2010), of size n1=200 has a proportion of p1=0.1.

The sample 2 (2011), of size n2=100 has a proportion of p2=0.15.

The difference between proportions is (p1-p2)=-0.05.

`p_d=p_1-p_2=0.1-0.15=-0.05`

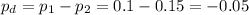
The pooled proportion, needed to calculate the standard error, is:

`p=(X_1+X_2)/(n_1+n_2)=(20+15)/(200+100)=(35)/(300)=0.1167`

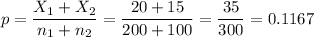
The estimated standard error of the difference between means is computed using the formula:

`s_(p1-p2)=\sqrt{(p(1-p))/(n_1)+(p(1-p))/(n_2)}=\sqrt{(0.1167*0.8833)/(200)+(0.1167*0.8833)/(100)}\\\\\\s_(p1-p2)=√(0.0005+0.001)=√(0.0015)=0.0393`

Then, we can calculate the z-statistic as:

`z=(p_d-(\pi_1-\pi_2))/(s_(p1-p2))=(-0.05-0)/(0.0393)=(-0.05)/(0.0393)=-1.27`

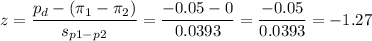
This test is a left-tailed test, so the P-value for this test is calculated as (using a z-table):

`P-value=P(z<-1.27)=0.102`

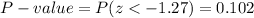
As the P-value (0.102) is bigger than the significance level (0.01), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that the proportion of college students who report having been diagnosed with depression has increased from 2010 to 2011.