Answer:
a) 0.277 = 27.7% probability that a randomly selected tyre lasts between 55,000 and 65,000 miles.
b) 0.348 = 34.8% probability that a randomly selected tyre lasts less than 48,000 miles.
c) 0.892 = 89.2% probability that a randomly selected tyre lasts at least 41,000 miles.
d) 0.778 = 77.8% probability that a randomly selected tyre has a lifetime that is within 10,000 miles of the mean
Explanation:
Problems of normally distributed distributions are solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by:
, the zscore of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
In this question, we have that:
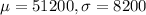
Probabilities:
A) Between 55,000 and 65,000 miles
This is the pvalue of Z when X = 65000 subtracted by the pvalue of Z when X = 55000. So
X = 65000

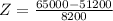
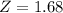
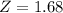 has a pvalue of 0.954
has a pvalue of 0.954
X = 55000

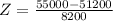
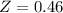
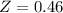 has a pvalue of 0.677
has a pvalue of 0.677
0.954 - 0.677 = 0.277
0.277 = 27.7% probability that a randomly selected tyre lasts between 55,000 and 65,000 miles.
B) Less than 48,000 miles
This is the pvalue of Z when X = 48000. So

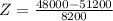

 has a pvalue of 0.348
has a pvalue of 0.348
0.348 = 34.8% probability that a randomly selected tyre lasts less than 48,000 miles.
C) At least 41,000 miles
This is 1 subtracted by the pvalue of Z when X = 41,000. So

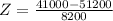

 has a pvalue of 0.108
has a pvalue of 0.108
1 - 0.108 = 0.892
0.892 = 89.2% probability that a randomly selected tyre lasts at least 41,000 miles.
D) A lifetime that is within 10,000 miles of the mean
This is the pvalue of Z when X = 51200 + 10000 = 61200 subtracted by the pvalue of Z when X = 51200 - 10000 = 412000. So
X = 61200


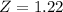
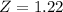 has a pvalue of 0.889
has a pvalue of 0.889
X = 41200

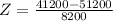

 has a pvalue of 0.111
has a pvalue of 0.111
0.889 - 0.111 = 0.778
0.778 = 77.8% probability that a randomly selected tyre has a lifetime that is within 10,000 miles of the mean