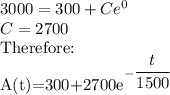Answer:
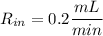
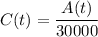
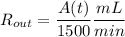
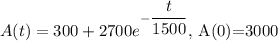
Explanation:
The volume of the swimming pool = 30,000 liters
(a) Amount of chlorine initially in the tank.
It originally contains water that is 0.01% chlorine.
0.01% of 30000=3000 mL of chlorine per liter
A(0)= 3000 mL of chlorine per liter
(b) Rate at which the chlorine is entering the pool.
City water containing 0.001%(0.01 mL of chlorine per liter) chlorine is pumped into the pool at a rate of 20 liters/min.
 (concentration of chlorine in inflow)(input rate of the water)
(concentration of chlorine in inflow)(input rate of the water)

(c) Concentration of chlorine in the pool at time t
Volume of the pool =30,000 Liter
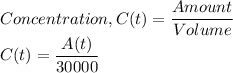
(d) Rate at which the chlorine is leaving the pool
 (concentration of chlorine in outflow)(output rate of the water)
(concentration of chlorine in outflow)(output rate of the water)
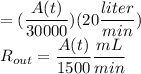
(e) Differential equation representing the rate at which the amount of sugar in the tank is changing at time t.
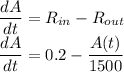
We then solve the resulting differential equation by separation of variables.
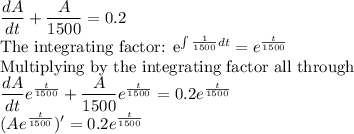
Taking the integral of both sides
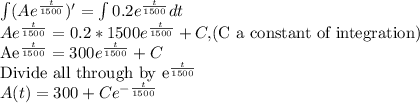
Recall that when t=0, A(t)=3000 (our initial condition)