Question

Find any points of discontinuity for the rational function. y = (x - 1)/(x ^ 2 - 2x - 8)

Answer 1

Asymptotic discontinuities at
`x = (-2)` and
`x = 4`.

Explanation:

A linear function has an asymptotic discontinuity at
`x = a` if
`(x - a)` is a factor of the denominator after simplification.

The numerator of this function,
`(x - 1)`, is linear in
`x`.

The denominator of this function,
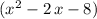`(x^(2) - 2\, x - 8)`, is quadratic in
`x`. Using the quadratic formula or otherwise, factor the denominator into binominals:

`\begin{aligned}y &= ((x - 1))/(x^(2) - 2\, x - 8) \\ &= ((x - 1))/((x - 4)\, (x + 2))\end{aligned}`.

Simplify the function by liminating binomials that are in both the numerator and the denominator.

Notice that in the simplified expression, binomial factors of the denominator are
`(x - 4)` and
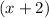`(x + 2)` (which is equivalent to
`(x - (-2))`.) Therefore, the points of discontinuity of this function would be
`x = 4` and
`x = (-2)`.