Question

Find the uncertainty in a calculated average speed from the measurements of distance and time. Average speed depends on distance and time according to this function v(t,x) = x/t. Your measured distance and time have the following values and uncertainties x = 8.1 meters, Δx = 0.9 meters and t = 1.7 seconds and Δt = 1.7 seconds. What is the uncertainty in the average speed, Δv ?

Answer 1

`(\Delta v)/(v)=0.426`

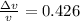
Explanation:

you have that the average sped is given by the following formula:

`v(x,t)=(x)/(t)`

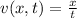
The uncertainty formula for a division is given by:

`(\Delta v)/(v)=\sqrt{((\Delta x)/(x))^2+((\Delta t)/(t))^2}` (1)

Δv: uncertainty in speed

Δx: uncertainty in the distance = 0.9m

Δt: uncertainty in time = 0.7s

x: distance = 8.1m

t: time = 1.7s

You replace the values of all parameters in the equation (1):

`(\Delta v)/(v)=\sqrt{((0.9)/(8.1))^2+((0.7)/(1.7))^2}\\\\(\Delta v)/(v)=0.426`

Hence, the relation between the uncertainty in the average velocity is 0.426