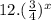Question

!!!PLEASE HELP!!!

the exponential function g, represented in the table, can be written as g(x)=a•b^x.

complete the equation for g(x).

please and thank you

Answer 1

g(x) =
`12.((3)/(4))^x`

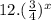
Explanation:

Exponential function g has been represented by the table attached,

Equation of the exponential function is,

g(x) =
`a.(b)^x`

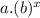
Now we substitute the values of x and g(x) from the table,

For a point (0, 12),

12 =
`a(b)^0`

a = 12

For another point (1, 9),

9 =
`a.(b)^1`

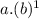
9 = a × b

9 = 12 × b

b =
`(9)/(12)`

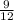
b =
`(3)/(4)`

Therefore, equation of the exponential function will be,

g(x) =
`12.((3)/(4))^x`