Answer:
The Net Present Value = $451180.733
Explanation:
Given that:
the initial cost of the project is $1670000
the initial increase in working capital is $198000
The total investment will be ; initial cost of the project + initial increase in working capital
The total investment = $1670000 + $198000
The total investment = $1868000
So, This investment cost is amortised to zero over four years; This implies that the project will depreciate in four years;
Now; lets determine the rate of the annual depreciation
The annual depreciation =
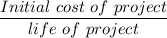
The annual depreciation =

The annual depreciation = $417500
Over to the annual operating cash flow; the annual operating cash flow can be calculated as follows:
annual operating cash flow = (sales - cost) × (1 - tax rate )+ (annual depreciation × tax rate )
Given that the tax rate = 21% = 0.21
annual operating cash flow = (1850000 -1038000)×(1-0.21)+(417500× 0.21)
annual operating cash flow = 812000 × 0.79 + 87675
annual operating cash flow = 641480 + 87675
annual operating cash flow = $729155
So; let's calculate the after tax salvage value for the project
We know that the sales values for the project in four years is $435,000 (i.e the disposal rate of the asset after four years)
Thus; the book value is zero
The after tax salvage value for the project can now be determined by the relation:
after tax salvage value = sale value - tax (sale value - book value)
after tax salvage value = 435000 - 0.21 (435,000 -0)
after tax salvage value = 435000 - 91350
after tax salvage value = $343650
Similarly; given that the recovering rate of the project is $198000;
The cash flow in year 4 will be: annual operating cash flow + after tax salvage + recovery working capital
cash flow in year 4 = $(729155 + 343650 + 198000)
cash flow in year 4 = $ 1270805
This implies that the cash flow from year one to three is : 729155
the cash flow in year four is : 1270805
Given that the Required return rate = 16.4% = 0.164
We can therefore determine the Present cash inflows by using the relation:

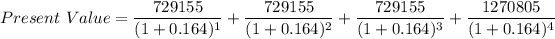
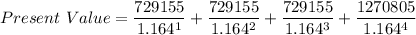
Present Value = $(626421.8213 + 538163.0767 + 462339.413+692256.4225)
Present Value = $2319180.733
Finally, The net present value = Present Value - Total Investment
Net Present Value = $(2319180.733 - 1868000)
Net Present Value = $451180.733
Therefore; The Net Present Value = $451180.733