Question

A survey in Men’s Health magazine reported that 39% of cardiologists said that they took vitamin E supplements. To see if this is still true, a researcher randomly selected 100 cardiologists and found that 36 said that they took vitamin E supplements. At α = 0.05, test the claim that 39% of the cardiologists took vitamin E supplements. A recent study said that taking too much vitamin e might be harmful how might this study make the results of the previous study invalid?

Answer 1

`z=\frac{0.36 -0.39}{\sqrt{(0.39(1-0.39))/(100)}}=-0.615`

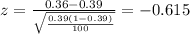
The p value for this case would be:

`p_v =2*P(z<-0.615)=0.539`

For this case since the p value is higher than the significance level we have enough evidence to FAIL to reject the null hypothesis and we can conclude that the true proportion is not different from 0.39

Explanation:

Information given

n=100 represent the random sample taken

X=36 represent the number of people that take E supplement

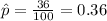`\hat p=(36)/(100)=0.36` estimated proportion of people who take R supplement

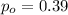`p_o=0.39` is the value that we want to test

`\alpha=0.05` represent the significance level

z would represent the statistic

`p_v` represent the p value

Hypothesis to test

We want to test if the true proportion is equatl to 0.39 or not, the system of hypothesis are.:

Null hypothesis:
`p=0.39`

Alternative hypothesis:
`p \\eq 0.39`

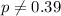
The statistic is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Replacing the info we got:

`z=\frac{0.36 -0.39}{\sqrt{(0.39(1-0.39))/(100)}}=-0.615`

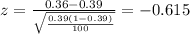
The p value for this case would be:

`p_v =2*P(z<-0.615)=0.539`

For this case since the p value is higher than the significance level we have enough evidence to FAIL to reject the null hypothesis and we can conclude that the true proportion is not different from 0.39