Question

Let C(q) represent the cost and R(q) represent the revenue, in dollars, of producing q items.

a. If C(50) = 4900 and C'(50) = 27, estimate C(52)
C(52)= $___________________

b. If C'(50) = 27 and R'(50) =37, approximately how much profit is earned by the 51st item?
The profit on the 51st itme is ____________________ dollars.

c. if C' (100) =41 and R'(100)= 37, should the company produce the 101st item?
The company _______________________ produce the 101st item

Answer 1

(a)$4954

(b)$10

(c)The company should not produce the 101st item.

Explanation:

(a)

`C'(50)=(C(52)-C(50))/(52-50) \\\\C(50) = 4900,C'(50) = 27\\\\$Therefore:\\27=(C(52)-4900)/(52-50)\\C(52)-4900=27*2\\C(52)=4900+54\\C(52)=\$4954`

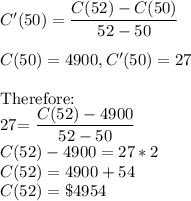
(b)If C'(50) = 27 and R'(50) =37

• Cost will increase by $27
• Revenue will increase by $37

Therefore, the profit earned on the 51st item

`=R'(50)-C'(50)`

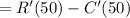
=37-27

=$10

(c)If C'(100) = 41 and R'(100) =37

• Cost will increase by $41
• Revenue will increase by $37

Therefore, the profit earned on the 101st item

Profit
`=R'(100)-C'(100)`

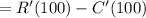
=37-41

=-$4

The company should not produce the 101st item. It would lose $4 if it does.