Question

The average retirement age for a certain country was reported to be 56.4 years according to an international group dedicated to promoting trade and economic growth. With the pension system operating with a​ deficit, a bill was introduced by the government during the summer to raise the minimum retirement age from 60 to 62. Suppose a survey of 40 retiring citizens is taken to investigate whether the new bill has raised the average age at which people actually retire. Assume the standard deviation of the retirement age is 55 years. Using α=0.10

Required:
Calculate the probability of a Type II error occurring if the actual population age is 57.5 years old.

Answer 1

|Z| = |-0.126| = 0.126 < 1.645

Null hypothesis is accepted

The retiring citizens is taken to investigate whether the new bill has raised the average age at which people actually retire.

μ = 57.5

Explanation:

Explanation:-

The average retirement age for a certain country was reported to be 56.4 years

The mean of the sample x⁻ = 56.4

The standard deviation of the Population 'σ'= 55 years

The mean of the population μ = 57.5

Null hypothesis: H₀:The retiring citizens is taken to investigate whether the new bill has raised the average age at which people actually retire.

μ = 57.5

Alternative Hypothesis : H₁: μ ≠57.5

Test statistic

`Z = (x^(-)-mean )/((S.D)/(√(n) ) )`

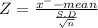
critical value:

`Z_{(\alpha )/(2) } = Z_(0.05) =1.645`

`Z = (56.4-57.5 )/((55)/(√(40) ) ) = -0.126`

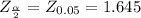
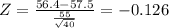
|Z| = |-0.126| = 0.126 < 1.645

The null hypothesis is accepted

Conclusion:-

The retiring citizens is taken to investigate whether the new bill has raised the average age at which people actually retire.

μ = 57.5