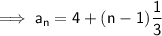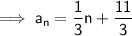Answer:
Arithmetic with common difference of
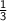
Explanation:
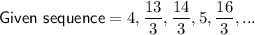
If a sequence is arithmetic, the difference between consecutive terms is the same (this is called the common difference).
If a sequence is geometric, the ratio between consecutive terms is the same (this is called the common ratio).
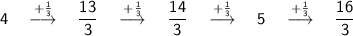
As the difference between consecutive terms is
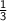 then the sequence is arithmetic with common difference of
then the sequence is arithmetic with common difference of
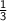
General form of an arithmetic sequence:
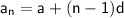
where:
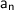 is the nth term
is the nth term- a is the first term
- d is the common difference between terms
Given:
- a = 4

So the formula for the nth term of this sequence is: