Answer:
a. 0.1024
b. 0.8976
Explanation:
The probability that x generators don't fail when they are needed follows a binomial distribution, because we have n identical and independent events (2 backup generators) with a probability p of success (1-0.32=0.68) and a probability q of failure (0.32).
So, the probability that x generator success are calculated as:
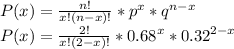
Then, the probability that both generators fail during a power outage is equal to the probability that 0 generators success. It is calculated as:
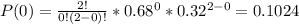
At the same way, the probability of having a working generator in the event of a power outage is equal to the probability that at least 1 generator success. It is calculated as:
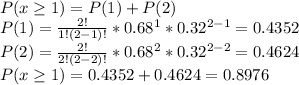
This probability is not high enough for the hospital, both generators fail approximately the 10% of the time when needed.