Question

A broth used to manufacture a pharmaceutical product has its sugar content, in mg/mL, measured several times on each of three successive days.

Day 1: 5.0, 4.8, 5.1, 5.1, 4.8, 5.1, 4.8, 4.8, 5.0, 5.2, 4.9, 4.9, 5.0
Day 2: 5.8, 4.7, 4.7, 4.9, 5.1, 4.9, 5.4, 5.3, 5.3, 4.8, 5.7, 5.1, 5.7
Day 3: 6.3, 4.7, 5.1, 5.9, 5.1, 5.9, 4.7, 6.0, 5.3, 4.9, 5.7, 5.3, 5.6
Can you conclude that the variability of the process is greater on the third day than on the second day?

Answer 1

Yes

Explanation:

To compare the variability of the measured sugar content for each day, we can estimate the standard deviation using the Range rule.

Standard Deviation =
`\frac{\text{Maximum Value-Minimum Value}}{4}`

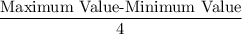
Day 1

5.0, 4.8, 5.1, 5.1, 4.8, 5.1, 4.8, 4.8, 5.0, 5.2, 4.9, 4.9, 5.0

• Maximum Value=5.2
• Minimum Value=4.8

Standard Deviation =
`(5.2-4.8)/(4)`

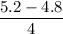
=0.1

Day 2

5.8, 4.7, 4.7, 4.9, 5.1, 4.9, 5.4, 5.3, 5.3, 4.8, 5.7, 5.1, 5.7

• Maximum Value=5.8
• Minimum Value=4.7

Standard Deviation =
`(5.8-4.7)/(4)`

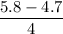
=0.275

Day 3

6.3, 4.7, 5.1, 5.9, 5.1, 5.9, 4.7, 6.0, 5.3, 4.9, 5.7, 5.3, 5.6

• Maximum Value=6.3
• Minimum Value=4.7

Standard Deviation =
`(6.3-4.7)/(4)`

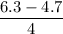
=0.4

Since the estimated standard deviation of the third day is higher than that of the second day, the variability of the process is greater on the third day than on the second day.