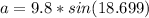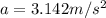Complete Question
A small block is released from rest at the top of a frictionless incline. The distance from the top of the incline to the bottom, measured along the incline, is 3.40 m. The vertical distance from the top of the incline to the bottom is 1.09 m. If
 , what is the acceleration of the block as it slides down the incline plane
, what is the acceleration of the block as it slides down the incline plane
Answer:
The acceleration is
Step-by-step explanation:
From the question we are told that
The distance from top to bottom of the inclined plane measured along the incline is
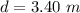
The distance from top to bottom of the inclined plane measured along the vertical axis is
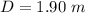
According to the SOHCAHTOA rule
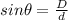
=>
![\theta = sin ^(-1) [(D)/(d) ]](https://img.qammunity.org/2021/formulas/physics/college/4266u34q7pl5rz0dc0jospqd7z2lgscv2q.png)
substituting values
=>
![\theta = sin ^(-1) [(1.09)/(3.40) ]](https://img.qammunity.org/2021/formulas/physics/college/zv6jyq3959e4fk8th9c0ftpm6tqtzxxjak.png)
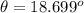 T
T
The acceleration of a block on a frictionless inclined plane is mathematically represented as
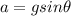
substituting values