Question

Predicting Election Results Throughout the US presidential election of 2012, polls gave regular updates on the sample proportion supporting each candidate and the margin of error for the estimates. This attempt to predict the outcome of an election is a common use of polls. In each case below, the proportion of voters who intend to vote for each candidate is given as well as a margin of error for the estimates. Indicate whether we can be relatively confident that candidate A would win if the election were held at the time of the poll. (Assume the candidate who gets more than of the vote wins.)

(a) Candidate A: 54% Candidate B: 46% Margin of error: ± 5% Confident A would win or Not confident in the outcome
(b) Candidate A: 52% Candidate B: 48% Margin of error: ± 1% Confident A would win or Not confident in the outcome
(c) Candidate A: 53% Candidate B: 47% Margin of error: ± 2% Confident A would win or Not confident in the outcome
(d) Candidate A: 58% Candidate B: 42% Margin of error: ± 10% Confident A would win or Not confident in the outcome

Answer 1

(a) Not confident.

(b) Confident A would win.

(c) Confident A would win.

(d) Not confident.

Explanation:

In order for us t be confident that Candidate A will win, Candidate A's percentage minus the margin of error must be higher than Candidate B's plus the margin of error.

(a)

`A = 54\%-5\%=49\%\\B = 46\%+5\%=51\%`

Not confident.

(b)

`A = 52\%-1\%=51\%\\B = 48\%+1\%=49\%`

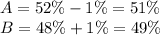
Confident A would win.

(c)

`A = 53\%-3\%=51\%\\B = 47\%+2\%=49\%`

Confident A would win.

(d)

`A = 58\%-10\%=48\%\\B = 42\%+10\%=52\%`

Not confident.