Answer:
a) The null and alternative hypothesis are:
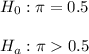
b) Sample proportion (p) = 0.6
Sample size (n) = 200
P-value = 0.0029
c) Conclusion: there is enough evidence to support the claim that the proportion of physicians over the age of 55 that have been sued at least once is significantly higher than 0.5.
Explanation:
The question is incomplete: there is no attached file with the data.
a) We want to test if more than half of the physiscians over the age of 55 have been sued at least once.
Then, the claim that will be stated in the alternative hypothesis should be that the proportion of physicians over the age of 55 that have been sued at least once is significantly higher than 0.5.
The null hypothesis should state that this proportion is not signficantly different from 0.5.
Then, the null and alternative hypothesis are:
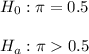
b) As we do not have the file, we will work with a sample with size n=200 and sample proportion of 0.6.
This sample proportion can be calculated from the data as
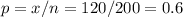
where x is the number of subjects in the sample that have been sued at least once.
The claim is that the proportion of physicians over the age of 55 that have been sued at least once is significantly higher than 0.5.
Then, the null and alternative hypothesis are:
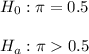
The significance level is 0.01.
The standard error of the proportion is:

Then, we can calculate the z-statistic as:
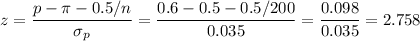
This test is a right-tailed test, so the P-value for this test is calculated as:

As the P-value (0.0029) is smaller than the significance level (0.01), the effect is significant.
The null hypothesis is rejected.
There is enough evidence to support the claim that the proportion of physicians over the age of 55 that have been sued at least once is significantly higher than 0.5.