Question

Suppose you work for the Department of Natural Resources and you want to estimate, with 95% confidence, the mean (average) length of all walleye fingerlings in a fish hatchery pond. You take a random sample of 36 fingerlings and determine that the average length is 7.4 inches and the population standard deviation is known to be 1.9 inches.

a. Construct a 95% confidence interval.
b. Interpret the Confidence Interval:
c. If the hatchery supervisor claimed that the average length of the walleye fingerlings fish is more than 7.5 inches, would you agree or disagree?

Answer 1

a)
`7.4-1.96(1.9)/(√(36))=6.78`

`7.4+1.96(1.9)/(√(36))=8.02`

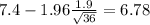
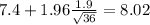
b) For this case we can conclude that at 95% of confidence the true mean for the lenght of all welleye fingerprints in a fish hatchery pond is between 6.78 and 8.02

c) For this case since the value of 7.5 is included in the confidence interval we don't have enough evidence to conclude that the true mean is actually higher than 7.5 inches

Explanation:

Information given

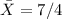`\bar X=7/4` represent the sample mean

`\mu` population mean (variable of interest)

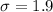`\sigma=1.9` represent the population standard deviation

n=36 represent the sample size

Part a

The confidence interval for the mean is given by :

`\bar X \pm z_(\alpha/2)(\sigma)/(√(n))` (1)

The Confidence is 0.95 or 95%, the significance is
`\alpha=0.05` and
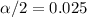`\alpha/2 =0.025`, and the critical value would be
`z_(\alpha/2)=1.96`

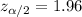
Replacing the info we got:

`7.4-1.96(1.9)/(√(36))=6.78`

`7.4+1.96(1.9)/(√(36))=8.02`

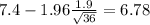
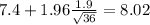
Part b

For this case we can conclude that at 95% of confidence the true mean for the lenght of all welleye fingerprints in a fish hatchery pond is between 6.78 and 8.02

Part c

For this case since the value of 7.5 is included in the confidence interval we don't have enough evidence to conclude that the true mean is actually higher than 7.5 inches