Question

Question

A manufacturer of home products is designing a new wall mount for large flat-panel televisions. Since some flat-panel
televisions weigh up to 100 pounds, the manufacturer wants to make sure that the mean weight of failure for the wall
mounts is greater than 180 pounds. Dayton is a researcher for the manufacturer who is conducting the test. He randomly
selects 35 wall mounts and tests them by using a machine that pulls down on the mounts until each one fails. The results
are shown in the data set provided. Based on the research completed during the development phase, Dayton assumes th
the population standard deviation is 6.9 pounds.
Use Excel to test whether the mean failure weight is greater than 180 pounds. Calculate the test statistic, z, rounding to t
decimal places, and the p-value, rounding to three decimal places.​

Answer 1

We conclude that the mean failure weight is greater than 180 pounds.

Explanation:

We are given that the manufacturer wants to make sure that the mean weight of failure for the wall mount is greater than 180 pounds. Assume that the population standard deviation is 6.9 pounds.

He randomly selects 35 wall mounts and tests them by using a machine that pulls down on the mounts until each one fails. The failure weights(in pounds) data is given as;

Failure weight (in pounds): 177, 171, 193, 186, 188, 175, 191, 190, 193, 184, 186, 190, 198, 201, 186, 195, 181, 189, 193, 195, 178, 194, 185, 187, 180, 192, 196, 194, 182, 183, 179, 188, 187, 181, 197.

Let
`\mu` = mean failure weight.

So, Null Hypothesis,
`H_0` :
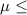`\mu \leq` 180 pounds {means that the mean failure weight is smaller than or equal to 180 pounds}

Alternate Hypothesis,
`H_A` :
`\mu` > 180 pounds {means that the mean failure weight is greater than 180 pounds}

The test statistics that would be used here One-sample z-test statistics as we know about population standard deviation;

T.S. =
`(\bar X-\mu)/((\sigma)/(√(n) ) )` ~ N(0,1)

where,
`\bar X` = sample mean weight =
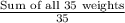`\frac{\text{Sum of all 35 weights}}{35}` = 187.57 pounds

`\sigma` = population standard deviation = 6.9 pounds

n = sample of wall mounts = 35

So, the test statistics =
`(187.57 - 180)/((6.9)/(√(35) ) )`

= 6.49

The value of the z-test statistic is 6.49.

Also, the P-value of the test statistics is given by;

P-value = P(Z > 6.49) = 1 - P(Z
`\leq` 6.49)

= 1 - 0.999 = 0.001

Since in the question we are not given with the level of significance so we assume it to be 5%. Now at the 5% significance level, the z table gives a critical value of 1.645 for the right-tailed test.

Since our test statistic is more than the critical value of z as 6.49 > 1.645, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.

Therefore, we conclude that the mean failure weight is greater than 180 pounds.