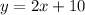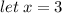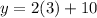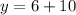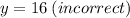Question

Find the gradient of the line 2y -8x = -2

Find the y-intercept of the line 3y=-15x -15
Does the point (3,15) lie on the line y=10+2x?
25 points to whoever answers all of them

Answer 1

1. gradient is 4.
2. y-intercept is -5.
3. No.

Explanation:

1. In order to find the gradient of equation, you have to make it into slope-form equation y = mx + b :

`2y - 8x = - 2`

`2y = 8x - 2`

`y = (8)/(2) x - (2)/(2)`

`y = 4x - 1`

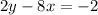
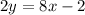
So m represents gradient. In this question, m is 4.

2. Make it into slope-form equation :

`3y = - 15x - 15`

`y = - (15)/(3) - (15)/(3)`

`y = - 5x - 5`

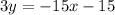
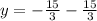
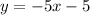
In this question, y-intercept is b which is -5.

3. You have to substitute x-coodinate into the equation to see whether it is equal to y-coordinate :

`y = 2x + 10`

`let \: x = 3`

`y = 2(3) + 10`

`y = 6 + 10`

`y = 16 \: (incorrect)`