Question

The given shape is a regular pentagon.

Work out the value of angle x and angle y.
E
B
D

Answer 1

Explanation:

Since there are missing data, let's discuss some facts about regular polygons and their angles.

1) The sum of its Interior angles is given by:

`S_i=180^(\circ)(n-2) \therefore \:for\: a\:pentagon\:S_i=180^(\circ)(5-2) =540^(\circ)`

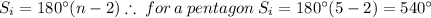
2) Since it is a regular pentagon, each interior angle is congruent:

Each interior angle:

`Interior\:angle=(540^(\circ))/(5) =108^(\circ)`

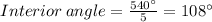
3) If we trace an outside line, the external angle will be supplemental, i.e.

`m\angle external= 180^(\circ)-108^(\circ) \therefore= 72^(\circ)`

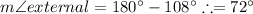
4) If we trace from each diagonal one line segment, in a regular pentagon that will form 3 triangles with one-third of 108º,i.e.,36º

As we can see, the in the picture below