Question

Find the missing lenghts of the sides

Answer 1

b = 8√3

c = 16

Explanation:

We cannot yet use the Pythagorean theorem because we only have one leg of the triangle

However, we can use trig identities to solve for the missing legs

I'll solve for b first so we can use the Pythagorean theorem after to find c

• We can use any of the trig identities to solve for b, but I will use the tangent identity since it involves the leg we were given
• 
  `tan(\alpha )=(opposite)/(adjacent)`
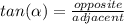
• Then solving for "opposite" which is our "b" will give us opposite = (adjacent)(tan(Ф))
• Plugging in our known values we get b = (8)(tan(60°)) = (8)(√3) = 8√3

Now that we have two legs of the triangle we can use the Pythagorean theorem to solve for the remaining leg, c.

• a² + b² = c² →
  `c=√(a^2+b^2)`
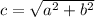
• Plugging in our two legs of the triangle we get
  `c=\sqrt{(8)^2+(8√(3) )^2}=√(64+192)=√(256)`
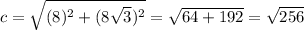
• c = 16

Answer 2

`b=8√(3), c=16`

Explanation:

Since the ratios of sides in a 30-60-90 triangle are
`1:√(3):2`, b must be
`8√(3)` and c must be 16. Hope this helps!