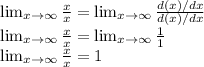Question

In calculus, the limit of x/x as x approaches infinity is 1. However, by the product rule, it would be the limit of x * limit of 1/x, which in turn evaluates to 0. Why is this so, and which one is wrong?

Answer 1

`\lim_(x \to \infty) (x)/(x)=1`

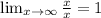
Calculating the limit by product rule is WRONG

Explanation:

Indeterminate Forms:

Indeterminate forms is an expression involving 2 functions whose limit cannot be determined by limits of individual functions. If we calculate the limits by general rules used for calculating the limits, we will not have a clear answer.

Examples of indeterminate forms are:

`(0)/(0), (\infty)/(\infty) , 0\cdot\infty, 1^(\infty),\infty-\infty, 0^0,\infty^0`

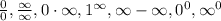
To find the limits of such forms, we have to use L'hospital rule, which states that if:

`\lim_(x \to \infty) (f(x))/(g(x)) = Indeterminate form \\ Then\\\lim_(x \to \infty) (f(x))/(g(x)) = \lim_(x \to \infty) (f'(x))/(g'(x))`

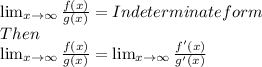
Solve the question:

`\lim_(x \to \infty) (x)/(x)= \lim_(x \to \infty) x\cdot \lim_(x \to \infty) (1)/(x) \\ \lim_(x \to \infty) (x)/(x)= =\infty\cdot(1)/(\infty) \\ \lim_(x \to \infty) (x)/(x)=\infty\cdot0`

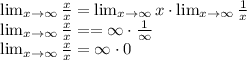
As it is an INDETERMINATE FORM, we cannot calculate its limit by product rule. We have to use L'Hospital Rule:

`\lim_(x \to \infty) (x)/(x)= \lim_(x \to \infty) (d(x)/dx)/(d(x)/dx)\\\lim_(x \to \infty) (x)/(x)=\lim_(x \to \infty) (1)/(1)\\\lim_(x \to \infty) (x)/(x)=1`