Question

A study was conducted on the critical-part failures from 36 NASCAR races. The researchers discovered that the time in hours until the first critical-part failure is exponentially distributed with a mean of 0.5 hour. Find the probability that the time until the first critical-part failure is more than 2 hours.

Answer 1

1.83% probability that the time until the first critical-part failure is more than 2 hours.

Explanation:

Exponential distribution:

The exponential probability distribution, with mean m, is described by the following equation:

`f(x) = \mu e^(-\mu x)`

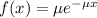
In which
`\mu = (1)/(m)` is the decay parameter.

The probability that x is lower or equal to a is given by:

`P(X \leq x) = \int\limits^a_0 {f(x)} \, dx`

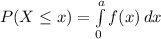
Which has the following solution:

`P(X \leq x) = 1 - e^(-\mu x)`

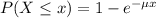
The probability of finding a value higher than x is:

`P(X > x) = 1 - P(X \leq x) = 1 - (1 - e^(-\mu x)) = e^(-\mu x)`

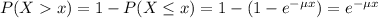
In this question, we have that:

`m = 0.5, \mu = (1)/(0.5) = 2`

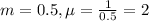
Find the probability that the time until the first critical-part failure is more than 2 hours.

`P(X > 2) = e^(-2*2) = 0.0183`

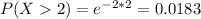
1.83% probability that the time until the first critical-part failure is more than 2 hours.