Question

What is the arc measure of \stackrel{\LARGE{\frown}}{AC}

AC


⌢


A, C, start superscript, \frown, end superscript on circle PPP in degrees?


^\circ


∘


degrees

Answer 1

it is 74

......................

Explanation:

Answer 2

72°

Explanation:

Given that the radius(r) of the circle is 10 units and the length of arc ABC is 16π

The length of arc ABC =
`(\theta)/(360)*2\pi r`

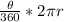
Where θ is the central angle in degrees.

Since the length of arc ABC is 16π,

`16\pi=(\theta)/(360)*2\pi r\\16\pi=(\theta)/(360)*2\pi *10\\\theta=(16\pi *360)/(2 \pi *10) \\\theta=288^0`

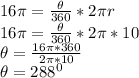
The angle in a circle = 360°, therefore:

Central angle for arc AB (θ) = 360 - Central angle for arc ABC = 360 - 288 = 72°

Therefore the arc measure of arc AB is 72°