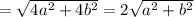Answer:
MN
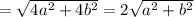
Explanation:
From the figure attached,
ΔMON is a right triangle and coordinates of the points M and N are M(0, 2b) and N(2a, 0).
Coordinates of midpoint P →
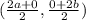
From the formula of the distance between two points,
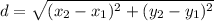
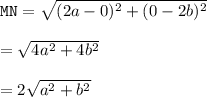
Similarly, OP
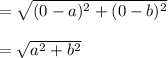
Therefore, OP
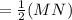
and MN