Answer:
A sample size of 79 is needed.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.
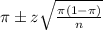
In which
z is the zscore that has a pvalue of
 .
.
For this problem, we have that:
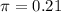
The margin of error is:
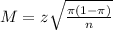
95% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
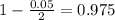 , so
, so
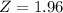 .
.
What sample size is needed if the research firm's goal is to estimate the current proportion of homes with a stay-at-home parent in which the father is the stay-at-home parent with a margin of error of 0.09?
A sample size of n is needed.
n is found when M = 0.09. So
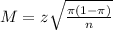
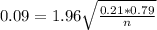
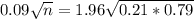
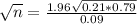

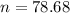
Rounding up to the nearest whole number.
A sample size of 79 is needed.