Answer:
Decay function, 70% per year.
Explanation:
A general exponential function is defined as
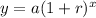 ...(1)
...(1)
where, a is initial value, |r| is rate of change and x is time in years.
If r>0, then it is a growth function and if r<0, then it is a decay function.
The given function is
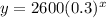
It can be rewritten as
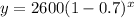
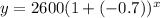 ...(2)
...(2)
On comparing (1) and (2), we get

Since r<0, therefore the given function is a decay function.

Therefore, the given function decreasing by 70% per year.