Question

Suppose Ball 1 is at a height of 19.6 m when it reaches the top of its trajectory. At the instant Ball 1 is at the top of its trajectory, Ball 2 is released from rest at the same height. How much time does it take for either ball to reach the ground? Note that the vertical component y of the ball’s displacement points downward, in the -y direction.

Answer 1

Both ball will take approximately 2 sec to reach the ground.

Step-by-step explanation:

Height reached by ball 1 at the top of its trajectory = 19.6 m

Assumptions:

• As ball 1 gets to the top of its trajectory, its velocity becomes zero.

• since not stated otherwise, we assume that both balls have the same properties

• If ball 2 is released at this instance, then they both will travel down at the same time interval and have initial velocity as zero
• since vertical travel is downwards, then acceleration due to gravity g is positive for both balls.

Using Newton's equation of motion,

s = ut +
`(1)/(2)`g
`t^(2)`

where s is the distance traveled down = 19.6 m

t is time taken to travel down = ?

g is acceleration due to gravity = 9.81
`ms^(-2)`

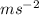
u is the initial velocity which is zero for both balls

substituting, we have

19.6 = (0 x t) +(
`(1)/(2)` x 9.81 x
`t^(2)`)

19.6 = 0 + 4.905
`t^(2)`

`t^(2)` = 3.996

t = 1.99 sec ≅ 2 sec