Answer:
Centre is (20, 0.05) and the radius is 9
Explanation:
General form of equation of a circle is expressed as
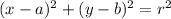 where r is the radius of the circle and (a, b) is the center of the circle.
where r is the radius of the circle and (a, b) is the center of the circle.
Given the equation of a circle to be
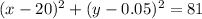 , rewriting the equation to conform to the general equation above we have;
, rewriting the equation to conform to the general equation above we have;
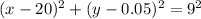
Comparing the equation given to the general equation above, a = 20, b = 0.05 and the radius of the circle will be 9. The center (a, b) will be (20, 0.05).