Answer:
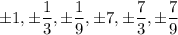 .
.
Explanation:
According to the Rational Root Theorem, the potential roots of a polynomial are
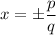
where, p is a factor of constant and q is a factor of leading term.
The given polynomial is
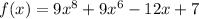
Here, 9 is the leading term and 7 is constant.
Factors of 9 are ±1, ±3, ±9.
Factors of 7 are ±1, ±7.
Using rational root theorem, the rational or potential roots are
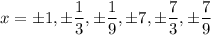
Therefore, the potential root of f(x) are
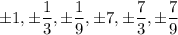 .
.