Question

A stationary submarine using sonar emits a 1080 Hz sound wave that reflects off of an object moving towards the sub. The reflected sound is mixed with the 1080 Hz sound and a beat frequency of 80 Hz is observed. The speed of sound in water is 1400 m/s. How fast is the object moving

Answer 1

v = 103.70 m/s

Step-by-step explanation:

To find the speed of the object, you first calculate the frequency of the reflected wave, by suing the information about the beat frequency:

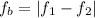`f_b=|f_1-f_2|` (1)

fb: frequency of the beat = 80Hz

f1: frequency of the submarine generated by the submarine = 1080Hz

By solving the equation (1) you have that f2 can take two values:

`f_2=1080Hz-80Hz=1000Hz\\f_2=1080Hz+80Hz=1160Hz`

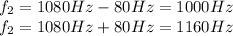
You use the second value (1160Hz) because the reflected wave comes from an object that is moving toward the sub.

Next, you use the formula for the Doppler effect's, for an object that is getting closer:

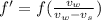`f'=f((v_w)/(v_w-v_s))` (2)

f': perceived frequency = 1160 Hz

f: frequency of the source = 1080 Hz

vw: speed of sound in water = 1400 m/s

vs: speed of the source = ?

You solve the equation (2) for vs, and you replace the values of the rest of the parameters:

`(v_w-v_s)f'=fv_w\\\\v_s=(v_w(f'-f))/(f)\\\\v_s=((1400m/s)(1160Hz-1080Hz))/(1080Hz)\\\\v_s=103.70(m)/(s)`

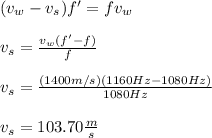
hence, the speed of the object that is moving toward the sub is 103.70 m/s