Question

The population of a town is 18,000. it decreases at a rate of 8% per year.In about how many years will the population be fewer than 11,000?

Answer 1

`11000= 18000 (1-0.08)^t`

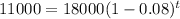
And solving for t we got:

`(11)/(18) = 0.92^t`

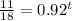
And if we apply natural logs we got:

`ln((11)/(18))= t ln (0.92)`

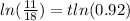
And then the value of t would be:

`t = 5.906`

So then after 5.906 or 6 years we will have approximately 11000 or less for the population

Explanation:

For this case we can use the following model:

`P(t) = A (1+r)^t`

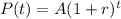
Where A = 18000 the initial value, r = -0.08 since is a decreasing rate and t the number of years. And we want to find the value of t until we have 11000 or lower and we can set up the following equation:

`11000= 18000 (1-0.08)^t`

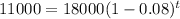
And solving for t we got:

`(11)/(18) = 0.92^t`

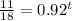
And if we apply natural logs we got:

`ln((11)/(18))= t ln (0.92)`

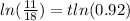
And then the value of t would be:

`t = 5.906`

So then after 5.906 or 6 years we will have approximately 11000 or less for the population