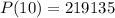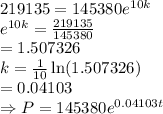Question

During the first decade of the century, the population of a certain city was 145,380 in 2000 and 219,135 in 2010. Find the exponential growth function that models the growth of the city. Use t = 0 to represent 2000, t = 10 to represent 2010, and so on ( round k to five decimal places)

Answer 1

The exponential growth function is
`P=145380e^(0.04103t)`

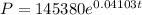
Explanation:

Given: The population of a certain city was 145,380 in 2000 and 219,135 in 2010.

To find: exponential growth function that models the growth of the city

Solution:

The exponential growth function is given by
`P=P_0 e^(kt)`

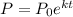
Here, P denotes total population after time t

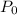`P_0` denotes initial population

k denotes rate of growth

t denotes time

As
`P(0)=145,380`,

`145380=P_0e^(0)\\145380=P_0\\P=145380e^(kt)`

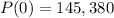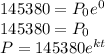
As
`P(10)=219135`

`219135=145380e^(10k)\\e^(10k)=(219135)/(145380)\\=1.507326\\k=(1)/(10)\ln (1.507326)\\=0.04103\\\Rightarrow P=145380e^(0.04103t)`