Question

Two runners in a marathon determine that the angles of elevation of a news helicopter covering the race are 38 degrees and 45 degrees. If the helicopter is 1700 feet above the finish line, how far apart are the runners? (Round your answer to the nearest foot) *

Answer 1

476 feet

Explanation:

The given problem when graphically depicted forms a right triangle which has been attached below.

We want to determine the distance between Runner A and Runner B in the diagram.

Using Trigonometric Ratios

In Right Triangle AHC

`\tan 38^\circ =(1700)/(AC) \\$Cross multiply$\\AC *\tan 38^\circ =1700\\AC =(1700)/(\tan 38^\circ)\\\\=2175.9$ feet`

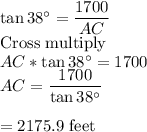
In Right Triangle BHC

`\tan 45^\circ =(1700)/(BC) \\$Cross multiply$\\BC *\tan 45^\circ =1700\\BC =(1700)/(\tan 45^\circ)\\\\=1700$ feet`

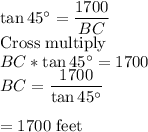
The distance between the two runners,

AB=AC-BC

=2175.9-1700

=475.9

=476 feet ( to the nearest foot)