Question

Fernando evaluated the expression below. StartFraction 5 (9 minus 5) over 2 EndFraction + (negative 2) (negative 5) + (negative 3) squared = StartFraction 5 (4) over 2 EndFraction minus 10 + 9 = StartFraction 20 over 2 EndFraction minus 10 + 9 = 10 minus 10 + 9 = 9. What was Fernando's error? Fernando evaluated the numerator of the fraction incorrectly. Fernando simplified StartFraction 20 over 2 EndFraction incorrectly. Fernando incorrectly found the product of –2 and –5. Fernando evaluated (negative 3) squared incorrectly.

Answer 1

this the correct answer Fernando incorrectly found the product of –2 and –5.

Step-by-step explanation:

Answer 2

Question:

Fernando evaluated the expression below.

`(5(9-5))/(2) + (-2)(-5) + (-3)^2 = (5(4))/(2) -10 + 9 = (20)/(2) - 10 + 9 = 10 - 10 + 9 = 9`

What was Fernando's error?

- Fernando evaluated the numerator of the fraction incorrectly.

- Fernando simplified 20/2 incorrectly.

- Fernando incorrectly found the product of –2 and –5.

- Fernando evaluated (-3)² incorrectly.

Answer:

- Fernando incorrectly found the product of –2 and –5.

Step-by-step explanation:

Given

`(5(9-5))/(2) + (-2)(-5) + (-3)^2 = (5(4))/(2) -10 + 9 = (20)/(2) - 10 + 9 = 10 - 10 + 9 = 9`

Required

Spot the error

The error in this evaluation is the product of -2 and -5 at step 2

When a negative number (-2) is multiplied by a negative number (-5), the outcome of the multiplication is always a positive number (10).

So, the result of -2 * -5 is 10 but Fernando incorrectly calculated it as -10.

Solving the expression, correctly

`(5(9-5))/(2) + (-2)(-5) + (-3)^2 = (5(4))/(2) + 10 + 9`

`(5(9-5))/(2) + (-2)(-5) + (-3)^2 = (20)/(2) + 10 + 9`

`(5(9-5))/(2) + (-2)(-5) + (-3)^2 = 10 + 10 + 9`

`(5(9-5))/(2) + (-2)(-5) + (-3)^2 = 29`

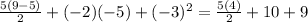
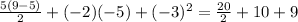
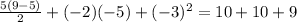
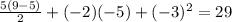
Hence, the actual result of the expression is 29 (not 9 as calculated by Fernando).