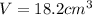Question

Find the volume of a pyramid with a square base, where the perimeter of the base is 8.2\text{ cm}8.2 cm and the height of the pyramid is 13\text{ cm}13 cm. Round your answer to the nearest tenth of a cubic centimeter.

Answer 1

`V=18.2cm^3`

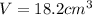
Explanation:

The volume of the pyramid is given by:

`V=(A_(b)h)/(3)`

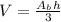
where
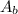`A_(b)` is the area of the base and
`h` is the height.

We know that the height is:

`h=13cm`

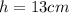
Thus we need to find the area of the square at the base.

The perimeter of the square is:

`p=8.2cm`

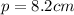
this means that the length of the sides of the square is :

`l=(p)/(4)\\ \\l=(8.2cm)/(4)\\ \\l=2.05cm`

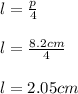
Now we can find the area of the base which the area of the square:

`A_(b)=l^2\\A_b=(2.05cm)^2\\A_b=4.2025cm^2`

and finally we find the volume:

`V=(A_(b)h)/(3)`

`V=((4.2025cm^2)(13cm))/(3)\\ \\V=(54.6325cm^3)/(3)\\ \\V=18.2108cm^3`

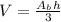
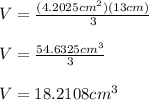
Which rounded to the nearest tenth of a cubic centimeter is:
`V=18.2cm^3`