Question

The tens digit is three less than the units digit. If the digits are reversed, the sum of the reversed number and the original number is 121. Find the original number.

Answer 1

47

Explanation:

MATH

Answer 2

Original number is 47

Explanation:

Given: The tens digit is three less than the units digit. If the digits are reversed, the sum of the reversed number and the original number is 121.

To find: the original number

Solution:

Let x denotes digit at ones place.

As the tens digit is three less than the units digit,

Digit at tens place =
`x-3`

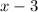
Original number =
`10(x-3)+x=11x-30`

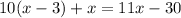
When digits are reversed,

Reversed number =
`10x+(x-3)=11x-3`

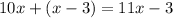
As the sum of the reversed number and the original number is 121,

`11x-30+11x-3=121\\22x-33=121\\22x=154\\x=(154)/(22)\\=7`

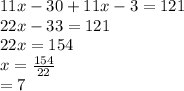
So,

original number =
`11x-30=11(7)-30=77-30=47`