Question

The table provides on road accidents reported to the police for the days in December

Accidents reported Frequency
0-5 9
6-10 10
11-15 6
16-20 6


Estimate the mean.
Give your answer rounded to 1 DP.

Answer 1

`Mean = 9.3`

Explanation:

Given

Let accidents reported be represented by x and the frequency be represented by f

x ----- f

0-5 -- 9

6-10 -- 10

11-15 -- 6

16-20 -- 6

Required

Calculate the Mean;

To calculate the mean of a grouped data, the first step is to calculate the midpoint of the observation;

When x = 0 - 5

Midpoint = 0.5(0 + 5) = 0.5 * 5 = 2.5

When x = 6 - 10

Midpoint = 0.5(6 + 10) = 0.5 * 16 = 8

When x = 11 - 15

Midpoint = 0.5(11 + 15) = 0.5 * 26 = 13

When x = 16 - 20

Midpoint = 0.5(16 + 20) = 0.5 * 36 = 18.

So, the new table is as follows;

x ------- f

2.5 ---- 9

8 ----- 10

13 ------ 6

18 ------ 6

Mean is calculated by ∑fx/∑f

Where ∑fx represent sum of the product of f and x

∑f represent sum of frequency

∑fx = 2.5 * 9 + 8 * 10 + 13 * 6 + 18 * 6

∑fx = 288.5

∑f = 9 + 10 + 6 + 6

∑f = 31

So,

`Mean =(288.5)/(31)`

`Mean = 9.3064516129`

`Mean = 9.3 (Approximated)`

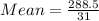
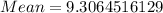
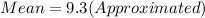
Hence, the mean of road accidents in December is 9.3