Question

Which statement is true about f(x) + 2 = 1/6 |x-3| ?

A) The graph of f(x) has a vertex of (–3, 2).
B) The graph of f(x) is a horizontal compression of the graph of the parent function.
C) The graph of f(x) opens downward.
D) The graph of f(x) has range of f(x) ≥ –2.

Answer 1

Option D.

Explanation:

Statement given,

f(x) + 2 =
`(1)/(6)|x - 3|`

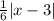
Or f(x) =
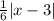`(1)/(6)|x-3|` -2

Parent function, g(x) = |x|

Absolute value function g(x) when shifted 3 units right,

g'(x) = |x - 3|

Vertically compressed by
`(1)/(6)` units and shifted 2 units down, then the new function will be

f(x) =
`(1)/(6)|x - 3|-2`

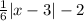
Characteristics of the graph of this function:

1). Vertex at (3, -2).

2). Vertical compression of the parent function by
`(1)/(6)`.

3). Graph opens upwards.

4). Range of the graph f(x) is f(x) ≥ -2.

Therefore, Option D will be the answer.