Answer:
a)
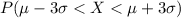
And from the empirical rule we know that this probability is 0.997 or 99.7%
b)
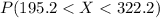
Using the z score we have:
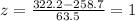
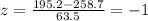
And within one deviation from the mean we have 68% of the values
Explanation:
For this case we defien the random variable of interest X as "blood platelet counts" and we know the following parameters:
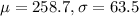
Part a
We can use the z score formula given by:
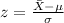
And we want this probability:
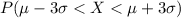
And from the empirical rule we know that this probability is 0.997 or 99.7%
Part b
For this case we want this probability:
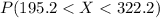
Using the z score we have:
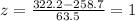
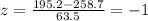
And within one deviation from the mean we have 68% of the values